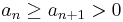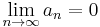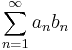Dirichlet's test

In mathematics, Dirichlet's test is a method of testing for the convergence of a series. It is named after mathematician Johann Dirichlet who published it in the Journal de Mathématiques Pures et Appliquées in 1862.^[1]

1 Statement
2 Proof
3 Applications
4 Notes
5 References
6 External links

Statement

The test states that if 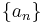$\{a_n\}$ is a sequence of real numbers and 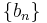$\{b_n\}$ a sequence of complex numbers satisfying

$a_n \geq a_{n%2B1} > 0$

$\lim_{n \rightarrow \infty} a_n = 0$

$\left|\sum^{N}_{n=1}b_n\right|\leq M$ for every positive integer N

where M is some constant, then the series

$\sum^{\infty}_{n=1}a_n b_n$

converges.

Proof

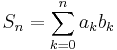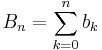Let $S_n = \sum_{k=0}^n a_k b_k$ and $B_n = \sum_{k=0}^n b_k$ .

From summation by parts, we have that 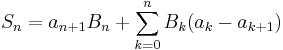$S_n = a_{n%2B1} B_n %2B \sum_{k=0}^n B_k (a_k - a_{k%2B1})$ .

Since 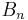$B_n$ is bounded by M and 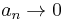$a_n \rightarrow 0$ , the first of these terms approaches zero, $a_{n%2B1}B_n \to 0$ as n→∞.

On the other hand, since the sequence 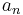$a_n$ is decreasing, 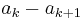$a_k - a_{k%2B1}$ is positive for all k, so 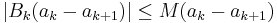$|B_k (a_k - a_{k%2B1})| \leq M(a_k - a_{k%2B1})$ . That is, the magnitude of the partial sum of B_n, times a factor, is less than the upper bound of the partial sum B_n (a value M) times that same factor.

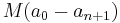But $\sum_{k=0}^n M(a_k - a_{k%2B1}) = M\sum_{k=0}^n a_k - a_{k%2B1}$ , which is a telescoping series that equals $M(a_0 - a_{n%2B1})$ and therefore approaches 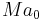$Ma_0$ as n→∞. Thus, 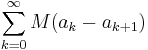$\sum_{k=0}^\infty M(a_k - a_{k%2B1})$ converges.

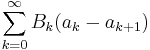In turn, $\sum_{k=0}^\infty |B_k(a_k - a_{k%2B1})|$ by the Direct Comparison test. The series $\sum_{k=0}^\infty B_k(a_k - a_{k%2B1})$ converges, as well, by the Absolute convergence test. Hence 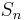$S_n$ converges.

Applications

A particular case of Dirichlet's test is the more commonly used alternating series test for the case

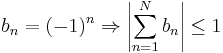$b_n = (-1)^n \Rightarrow\left|\sum_{n=1}^N b_n\right| \leq 1$ .

Another corollary is that 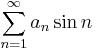$\sum_{n=1}^\infty a_n \sin n$ converges whenever 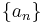$\{a_n\}$ is a decreasing sequence that tends to zero.

Notes

^ Démonstration d’un théorème d’Abel. Journal de mathématiques pures et appliquées 2nd series, tome 7 (1862), p. 253-255.

References

Hardy, G. H., A Course of Pure Mathematics, Ninth edition, Cambridge University Press, 1946. (pp. 379-380).

Voxman, William L., Advanced Calculus: An Introduction to Modern Analysis, Marcel Dekker, Inc., New York, 1981. (§8.B.13-15) ISBN 0-8247-6949-X.

External links

Proof at PlanetMath.org